|

Here's what didn't happen on Sept. 10th:
The world did not end. Switching on
the world's largest and most powerful particle
accelerator near Geneva, Switzerland, did not
trigger the creation of a microscopic black
hole. And that black hole did not start rapidly
sucking in surrounding matter faster and faster
until it devoured the Earth, as sensationalist news
reports had suggested it might.
Of course, because you're alive and
reading this article today, you already knew that.
Currently the accelerator, an underground ring 5
miles across called the Large Hadron Collider (LHC),
has been shut down for repairs. But once the
immensely powerful machine starts back up, is there
a chance that the doomsday scenario could still
occur?
Relax. As Mark Twain might have
said, reports of Earth's death have been greatly
exaggerated.
"There never really was a danger
from the accelerator, but that sure didn't stop
people from speculating that there might be!" says
Robert Johnson, a physicist at the Santa Cruz
Institute for Particle Physics and a member of the
science team for NASA's Fermi Gamma-ray Space
Telescope, which launched in June to study gamma
rays from many phenomena, including possible
evaporating black holes.
There are several reasons why the
world did not come to an end on Sept. 10th, and why
the Large Hadron Collider isn't capable of
triggering such a calamity.
First of all, yes, it is true that
the LHC might create microscopic black holes. But,
for the record, it could not have created one on its
first day. That's because the physicists at CERN
didn't steer beams of protons into each other to
create high-energy collisions. Sept. 10th was just a
warmup run. To date, the collider still has not
produced any collisions, and it is the extreme
energy of those collisions — up to 14 tera-electron
volts — that could potentially create a microscopic
black hole.
Actually, once the LHC is running
again and begins producing collisions, physicists
will be ecstatic if it creates a tiny black hole. It
would be the first experimental evidence to support
an elegant but unproven and controversial "theory of
everything" called string theory.
In string theory, electrons,
photons, quarks, and all the other fundamental
particles are different vibrations of infinitesimal
strings in 10 dimensions: 9 space dimensions and one
time dimension. (The other 6 space dimensions are
hidden by one explanation or another, for example by
being "curled up" on an extremely small scale.) Some
physicists tout string theory's mathematical
elegance and its ability to integrate gravity with
the other forces of nature. The widely accepted
Standard Model of particle physics does not include
gravity, which is one reason why it does not predict
that the LHC would create a gravitationally
collapsed point — a black hole — while string theory
does.
Many physicists have started to
doubt whether string theory is true. But assuming
for a moment that it is, what would happen when a
black hole is born inside the LHC? The surprising
answer is "not much." Even if the black hole
survives for more than a fraction of a second (which
it probably wouldn't), most likely it would be flung
out into space. "It would only have the mass of a
hundred or so protons, and it would be moving at
near the speed of light, so it would easily have
escape velocity," Johnson explains. Because the tiny
black hole would be less than a thousandth the size
of a proton and would have an exceedingly weak
gravitational pull, it could easily zip through
solid rock without ever touching — or sucking in —
any matter. From the perspective of something this
tiny, the atoms that make up "solid" rock appear to
be almost entirely empty space: the vast space
between the atoms' nuclei and their orbiting
electrons. So a micro black hole could shoot down
through the center of the Earth and out the other
side without causing any damage just as easily as it
could shoot up through 300 feet of the Swiss
countryside. Either way, it would end up out in the
near-vacuum of space, where the odds of it touching
and sucking in any matter so that it could grow into
a menace would be smaller still.
So the first thing a micro-black
hole would do is leave the planet safely behind. But
there are other, even stronger reasons why
scientists believe the LHC poses no threat to Earth.
For one, a black hole created in the LHC would
almost certainly evaporate before it got very far,
most scientists believe. Stephen Hawking, the
physicist who wrote A Brief History of Time,
predicted that black holes radiate energy, a
phenomenon known as Hawking radiation. Because of
this steady loss of energy, black holes eventually
evaporate. The smaller the black hole, the more
intense the Hawking radiation, and the quicker the
black hole will vanish. So a black hole a thousand
times smaller than a proton should disappear almost
instantly in a quick burst of radiation.

"Hawking's
prediction is not based on speculative string theory
but rather on well understood principles of quantum
mechanics and particle physics," Johnson notes.

Despite its strong theoretical foundations, Hawking
radiation has never been observed directly. Still,
scientists are confident that any black hole created
by the LHC would pose no threat. How can they be so
sure? Because of cosmic rays. Thousands of times per
day, high-energy cosmic rays strike the Earth's
atmosphere, colliding with molecules in the air with
at least 20 times more energy than the most powerful
collisions that the LHC can produce. So if this new
accelerator could make Earth-devouring black holes,
cosmic rays would have already done so billions of
times during Earth's long history.
And yet, here we are. Let the collisions begin!
Science @
NASA
Source: http://www.redorbit.com/news/science/1586017/the_day_the_world_didnt_end
LHC Images - click on thumbnail for
larger image
[Full CERN collection here]

Tunnel Schematic

Tunnel for real - sector 81

Tunnel for real - sector 81
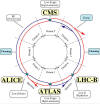
Overview schematic
shows the four main experiments and the two ring
structure of the LHC
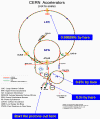
Overview of CERN's acclerator layout

The key element - the 1232 dipoles bend
the beam around te 27 km circumference

Joining things up
Once in place the magnet have to be connect - a very
complicated task.

Views of tunnel - mostly empty!
3d images from Peter McCready (Atlas
and the ring)

Source: http://lhc-machine-outreach.web.cern.ch/lhc-machine-outreach/lhc_in_pictures.htm
Micro Black Holes
Black holes are fascinating! They merge
together completely different fields of physics: From General
Relativity overthermodynamics and quantum
field theory, they
do now also reach into the regime of particle and
collider physics.

As explained in the intro about extra
dimensions, in the presence of additional
large compactified dimensions, it would be possible to
produce tiny black holes at future colliders. In this
case, we would be able to experimentally test Planck
scale physics and the onset of quantum gravity with the Large
Hadron Collider (LHC), which is scheduled
to start next summer. The formation of black holes is a
fairly robust prediction and one of the most general
expectations that one can have, even though the details
are still subject to research. For me, it is quite
amazing to see how this field has evolved during the
last decade. Starting from a smiled upon speculation, it
has by now become a widely accepted scenario for physics
beyond the standard model, which is included in
simulations of LHC events.
1. Micro Black Holes in Large Extra
Dimensions
In the standard 3+1 dimensional
space-time, the production of black holes requires a
concentration of energy-density which can not be reached
in the laboratory. But in a higher dimensional
space-time, gravity becomes stronger at small distances
and therefore the
event horizon is
located at a larger radius. This radius can be so large
that we could bring particles closer together than their
horizon. A black hole could be created.
The presence of extra dimensions
results in a modification of the predictions of the
standard model, which become important from a certain
energy scale 'the new fundamental scale', and which
might be accessible at the LHC. Due to the Heisenberg
uncertainty, it
requires a large energy to get particles into a small
volume. Only energies close by the new fundamental scale
would be sufficient to produce a black hole out of this
same energy. For collider physics one is therefore
interested in the case where the black hole has a mass
close to the new fundamental scale. This corresponds to
a horizon radius close to the inverse of the new
fundamental scale, which is much much smaller than the
radius of the extra dimensions. To a good approximation,
this tiny black hole just does not notice that the extra
dimensions are compactified, and one can neglect the
boundary condition. (The
higher dimensional Schwarzschild-metric
for this case has been derived by Myers
and Perry in '86)
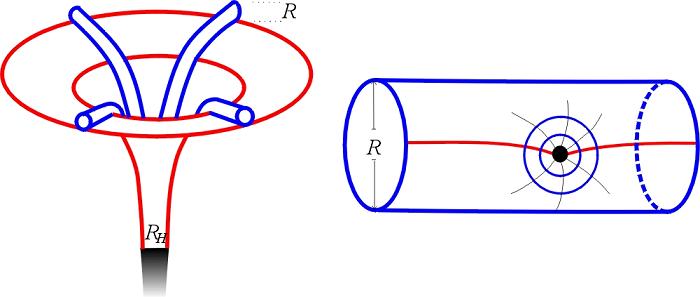
On the other hand, for astrophysical
objects we expect to find back the usual 3-dimensional
description. In this case, the horizon radius is much
larger than the radius of the extra dimensions and the
influence of the extra dimensions is negligible. Those
two case are depicted in the figure below. We will be
interested in the case depicted on the right side. R is
the radius of the extra dimensions (all of them have the
same radius) and RH is
the horizon radius of the black hole
2. Production of Black
Holes
Let us consider two elementary
particles, approaching each other with a very high
kinetic energy in the center-of-mass system close to the
new fundamental scale. At those high energies, the
particles can come very close to each other since their
high energy allows a tightly packed wave package despite
the uncertainty relation. If the impact parameter is
small enough, which will happen to a certain fraction of
the particles, we have the two particles plus their
large kinetic energy in a very small region of space
time. If the region is smaller than the Schwarzschild
radius connected with the energy of the partons, the
system will collapse and form a black hole.
The production of a
black hole in a high energy collision is probably the
most inelastic process one might think of. Since the
black hole is not an ordinary particle of the standard
model, and its correct quantum theoretical treatment is
unknown, it is commonly treated as a metastable state,
which is produced and decays according to the
semi-classical formalism of black hole physics. To
compute the production details, the cross-section of the
black holes can be approximated by the classical
geometric cross-section Pi R2. A common
approach to improve the naive picture of colliding point
particles is to treat the creation of the horizon as a
collision of two shock fronts in an Aichelburg-Sexl
geometry describing the fast moving particles.
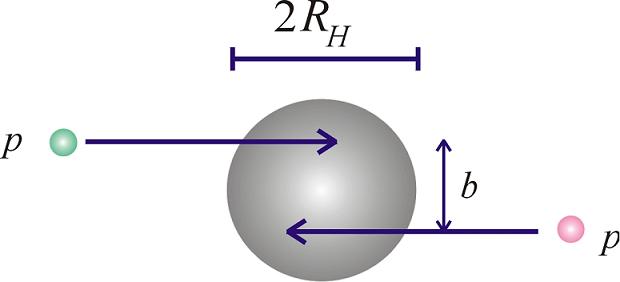
Looking at the figure on the left, we
also see that, due to conservation laws, the angular
momentum of the formed object only vanishes in
completely central collisions with zero impact
parameter. In the general case, we will have an angular
momentum, and the black hole might also carry an
electric charge.
Another assumption which goes into the
production details is the existence of a threshold for
the black hole formation. From general relativistic
arguments, two point like particles in a head on
collision with zero impact parameter (the b in
the figure above) will always form
a black hole, no matter how large or small their energy.
At small energies however, we expect this to be
impossible due to the smearing of the wave functions by
the uncertainty relation. This then results in a
necessary minimal energy to allow for the required close
approach. This threshold is of order of the new
fundamental scale, though the exact value is unknown
since quantum gravity effects should play an important
role for the wave functions of the colliding particles.
Using the geometrical cross section formula, it is now
possible to compute the differential and total cross
sections for black hole production. This also allows us
to estimate the total number of black holes, that would
be created at the LHC per year. Inserting the expected
technical details for the collider, one finds a number
of approximately 109 created
black holes per year! This means, about one black hole
per second.
3. Evaporation of Black
Holes
It was shown by
Hawking in '75 that
a black hole emits particles with a temperature that is
inverse to its mass. This means, the smaller the black
hole, the hotter it will be. Since we are talking about
really tiny black holes, they are very hot. The typical
temperature of the micro black holes is about 200 GeV or
1016 Kelvin!
The evaporation rate (massloss per time) of the higher
dimensional black hole can be computed using the
thermodynamics of black holes. Once produced, the black
holes will undergo an evaporation process whose thermal
properties carry information about the number and the
radius of the extra dimension. An analysis of the
evaporation will therefore offer the possibility to
extract knowledge about the topology of our space time
and the underlying theory. The evaporation process can
be categorized in three characteristic stages:
1. Balding
phase: In
this phase the black hole radiates away the multipole
moments it has inherited from the initial configuration,
and settles down in a hairless state. During this stage,
a certain fraction of the initial mass will be lost in
gravitational radiation.
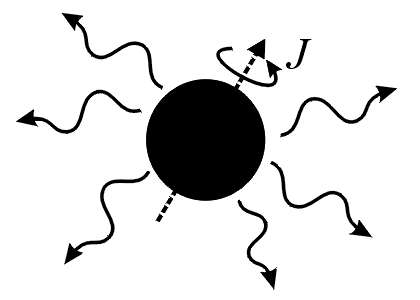
2. Evaporation
phase: The
evaporation phase starts with a spin down phase in which
the Hawking radiation carries away the angular momentum,
after which it proceeds with emission of thermally
distributed quanta until the black hole reaches Planck
mass. The radiation spectrum contains all Standard Model
particles, which are emitted on our brane, as well as
gravitons, which are also emitted into the extra
dimensions. It is expected that most of the initial
energy is emitted in during this phase in Standard Model
particles.
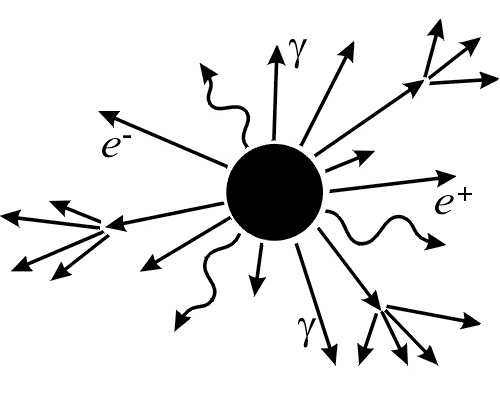
3. Planck
phase: Once
the black hole has reached a mass close to the Planck
mass, it falls into the regime of quantum gravity and
predictions become increasingly difficult. It is
generally assumed that the black hole will either
completely decay in some last few Standard Model
particles or a stable remnant will be left, which
carries away the remaining energy.
To perform a realistic simulation of the
evaporation process, one has to take into account the
various particles of the standard model with the
corresponding degrees of freedom and spin statistics. In
the extra dimensional scenario, standard model particles
are bound too our submanifold whereas the gravitons are
allowed to enter all dimensions. For a precise
calculation one also has to take into account that the
presence of the gravitational field will modify the
radiation properties for higher angular momenta through
backscattering at the potential well. These energy
dependent greybody factors can be calculated by
analyzing the wave equation in the higher dimensional
spacetime and the arising absorption coefficients. A
very thorough description of these evaporation
characteristics has been given by Kanti
in 2004 which
confirms the expectation that the bulk/brane evaporation
rate is of comparable magnitude but the brane modes
dominate.
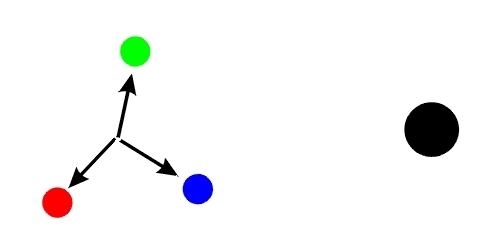
One of the primary observables in high
energetic particle collisions is the transverse momentum
of the outgoing particles, pT(pee-tee),
the component of the momentum transverse to the
direction of the beam. Two colliding partons with high
energy can produce a pair of outgoing particles, moving
in opposite directions with high pT but
carrying a color charge, as depicted in the figure to
the right.
Due to the quark
confinement, the color has to be neutralized. This
results in a shower of several bound states, the
hadrons, which includes mesons (consisting
of a quark and an antiquark, like the pions) as well as baryons (consisting
of three quarks, like the neutron or the proton). The
number of these produced hadrons and their energy
depends on the energy of the initial partons. This
process will cause a detector signal with a large number
of hadrons inside a small opening angle. Such an event
is called a jet.
Typically these jets come in pairs of
opposite direction. A smaller number of them can also be
observed with three or more outgoing showers. This
observable will be strongly influenced by the production
of black holes. To understand the signatures that are
caused by the black holes we have to examine their
evaporation properties. As we have seen before, the
smaller the black hole, the larger is its temperature
and so, the radiation of the discussed tiny black holes
is the dominant signature caused by their presence. The
high temperature results in a very short lifetime such
that the black hole will decay close by the collision
region and can be interpreted as a metastable
intermediate state.
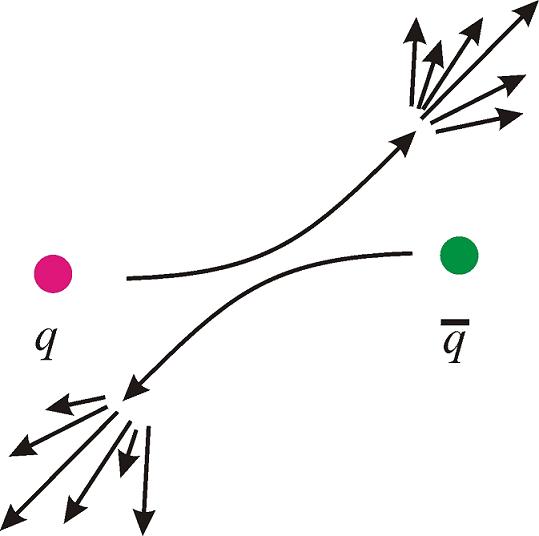
Due to the high energy captured in the
black hole, the decay of such an object is a very
spectacular event with a distinct signature. The number
of decay products, the multiplicity, is high compared to
standard model processes and the thermal properties of
the black hole will yield a high sphericity of the
event. Furthermore, crossing the threshold for black
hole production causes a sharp cut-off for high
energetic jets as those jets now end up as black holes
instead, and are re-distributed into thermal particles
of lower energies. Thus, black holes will give a clear
signal. A schematic picture of this process is shown on
the left.
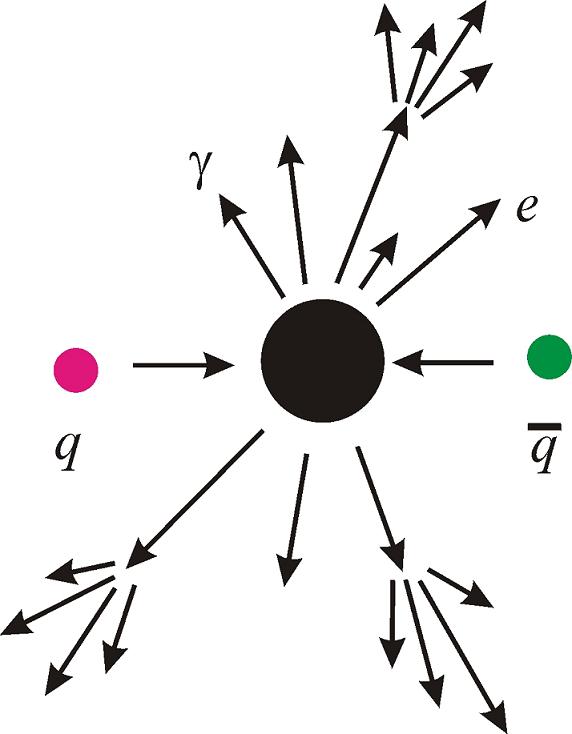
It is apparent that the consequences of
black hole production are quite disastrous for the
future of collider physics! Once the collision energy
crosses the threshold for black hole production, no
further information about the structure of matter at
small scales can be extracted. As it was put by Giddings
and Thomas, this would be ''the
end of short distance physics''
By now, several experimental groups
include black holes into their search for physics beyond
the standard model. Ideally, the energy distribution of
the decay products allows a determination of the
temperature (by fitting the energy spectrum to the
predicted shape) as well as of the total mass of the
object (by summing up all energies). This then allows to
reconstruct the fundamental scale, and the number of
extra dimensions. The quality of the determination
depends on the uncertainties in the theoretical
prediction as well as on the experimental limits e.g.
background from standard model processes. Besides the
formfactors of black hole production and the greybody
factors of the evaporation, the largest theoretical
uncertainties turnout to be the final decay and the time
variation of the temperature. In case the black hole
decays very fast, it can be questioned whether it has
time to readjust its temperature at all or whether it
essentially decays completely with its initial
temperature. Also, the determination of the properties
depends on the number of emitted particles. The less
particles, the more difficult the analysis
However, in my opinion the most crucial
uncertainty are the latest stages of the evaporation.
For hadron colliders like the LHC, the last stages with
black hole masses close by the production threshold will
dominate the signature, since most of the black holes
are actually produced out of parton collisions with a
total center-of-mass energy close by even this
threshold. In hadronic collisions there are thus very
little black holes which actually capture the total
available energy of 14 TeV, since the proton's energy
gets distributed on its constituents. Such a problem
would not be present for a lepton collider.
Source:
|

